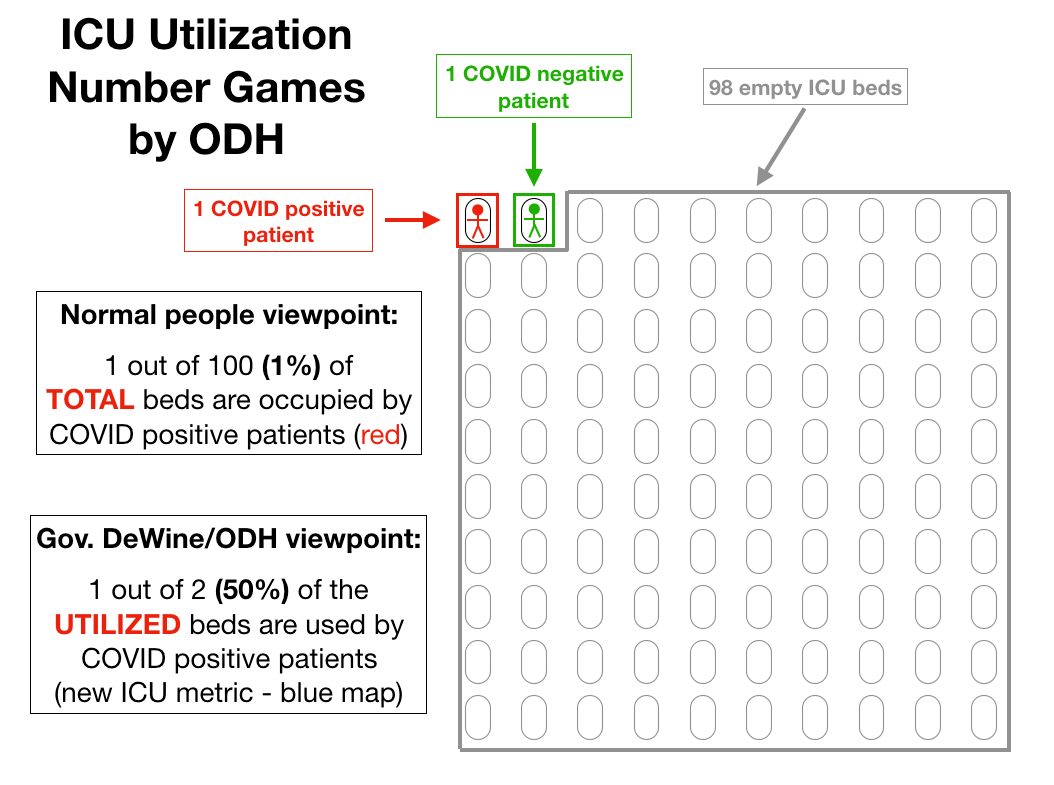
Algebra 1 and pre-algebra worksheets are something that all would love to have been able to dominate in high school. Sometimes they do not dominate it there and that's when it could be a bit difficult.
More from Arthouse-Kuklite
More from Maths
OK, I may be guilty of a DoS attack attempt on mathematicians' brains here, so lest anyone waste too much precious brain time decoding this deliberately cryptic statement, let me do it for you. •1/15
First, as some asked, it is to be parenthesized as: “∀x.∀y.((∀z.((z∈x) ⇒ (((∀t.((t∈x) ⇒ ((t∈z) ⇒ (t∈y))))) ⇒ (z∈y)))) ⇒ (∀z.((z∈x) ⇒ (z∈y))))” (the convention is that ‘⇒’ is right-associative: “P⇒Q⇒R” means “P⇒(Q⇒R)”), but this doesn't clarify much. •2/15
Maybe we can make it a tad less abstruse by using guarded quantifiers (“∀u∈x.(…)” stands for “∀u.((u∈x)⇒(…))”): it is then “∀x.∀y.((∀z∈x.(((∀t∈x.((t∈z) ⇒ (t∈y)))) ⇒ (z∈y))) ⇒ (∀z∈x.(z∈y)))”. •3/15
Maybe a tad clearer again by writing “P(u)” for “u∈y” and leaving out the quantifier on y, viꝫ: “∀x.((∀z∈x.(((∀t∈x.((t∈z) ⇒ P(t)))) ⇒ P(z))) ⇒ (∀z∈x.P(z)))” [✯]. Now it appears as an induction principle: namely, … •4/15
… “in order to prove P(z) for all z∈x, we can assume, when proving P(z), that P(t) is already known for all t∈z∩x” (n.b.: “(∀z.(Q(z)⇒P(z)))⇒(∀z.P(z))” can be read “in order to prove P(z) for all z, we can assume Q(z) known when proving P(z)”). •5/15
\u2200x.\u2200y.((\u2200z.((z\u2208x) \u21d2 ((\u2200t.((t\u2208x) \u21d2 (t\u2208z) \u21d2 (t\u2208y)))) \u21d2 (z\u2208y))) \u21d2 (\u2200z.((z\u2208x) \u21d2 (z\u2208y))))
— Gro-Tsen (@gro_tsen) February 12, 2021
First, as some asked, it is to be parenthesized as: “∀x.∀y.((∀z.((z∈x) ⇒ (((∀t.((t∈x) ⇒ ((t∈z) ⇒ (t∈y))))) ⇒ (z∈y)))) ⇒ (∀z.((z∈x) ⇒ (z∈y))))” (the convention is that ‘⇒’ is right-associative: “P⇒Q⇒R” means “P⇒(Q⇒R)”), but this doesn't clarify much. •2/15
Maybe we can make it a tad less abstruse by using guarded quantifiers (“∀u∈x.(…)” stands for “∀u.((u∈x)⇒(…))”): it is then “∀x.∀y.((∀z∈x.(((∀t∈x.((t∈z) ⇒ (t∈y)))) ⇒ (z∈y))) ⇒ (∀z∈x.(z∈y)))”. •3/15
Maybe a tad clearer again by writing “P(u)” for “u∈y” and leaving out the quantifier on y, viꝫ: “∀x.((∀z∈x.(((∀t∈x.((t∈z) ⇒ P(t)))) ⇒ P(z))) ⇒ (∀z∈x.P(z)))” [✯]. Now it appears as an induction principle: namely, … •4/15
… “in order to prove P(z) for all z∈x, we can assume, when proving P(z), that P(t) is already known for all t∈z∩x” (n.b.: “(∀z.(Q(z)⇒P(z)))⇒(∀z.P(z))” can be read “in order to prove P(z) for all z, we can assume Q(z) known when proving P(z)”). •5/15
In light of my tweet thread about the category of finite sets and commutative monoids (https://t.co/jnY0wZZbxq), I thought I might try to say what the analogue is for braided monoidal things (although much of this is still somewhat hypothetical).
It's also just kind of a cool combinatorial structure! I've been talking to @CreeepyJoe about this lately, as well as @grassmannian.
The first thing you have to know is that, in a braided monoidal category you can still have commutative monoids. Since a braided monoidal category C has a "twist" map for every object β(x):x⊗x→x⊗x, if x is a monoid you can ask for the following diagram to commute:
Remember that being symmetric monoidal just means that if you take the twist map above and do it twice, you get the identity map, but braided monoidal doesn't mean that. But it's okay! You can still define commutative monoids here.
But so anyway, we can talk about commutative monoids in braided monoidal categories.
So okay, here's a thread on the category of finite sets and a way in which it controls algebraic structure in symmetric monoidal categories. I think it's some really pretty stuff.
— Jonathan Beardsley (@JBeardsleyMath) December 6, 2020
It's also just kind of a cool combinatorial structure! I've been talking to @CreeepyJoe about this lately, as well as @grassmannian.
The first thing you have to know is that, in a braided monoidal category you can still have commutative monoids. Since a braided monoidal category C has a "twist" map for every object β(x):x⊗x→x⊗x, if x is a monoid you can ask for the following diagram to commute:
Remember that being symmetric monoidal just means that if you take the twist map above and do it twice, you get the identity map, but braided monoidal doesn't mean that. But it's okay! You can still define commutative monoids here.
But so anyway, we can talk about commutative monoids in braided monoidal categories.
